

Find the first three terms in the expansion of:
\((4a - 5b)^6\)
\(=4096a^6 - 30720a^5b \\+96000a^4b^2 ...\)
If £200 is invested with an interest rate of 3% compounded quarterly, find the value of the investment after 5 years. £232.24
Here are the coordinates of 3 vertices of a square, what are the coordinates of the 4th?
\((1,1),(6,6),(-4,6)\)
(1,11)
\( X \sim N(-25, 3^2)\)
Find
\( P(-20\lt X \lt-10) \)
\(0.0478\)
Factorise:
\(x^2-1\)
\((x+1)(x-1)\)
Factorise:
\(6x^2-x-1\)
\((3x+1)(2x-1)\)
Draw a rough sketch of the graph of:
\(y=2x-2\)
Gradient 2
y intercept -2
What is the value of:
\(3^{0}\)
\(= 1\)
Find angle BCA if AB = 4.6m and BC = 6.6m. 44.2o
Find AC if angle ABC = 55o and BC = 3.2m. 2.62m
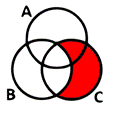
Describe the red region.
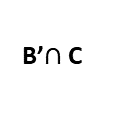
\(y = 4x^3 - 4x^2 + 6x\)
Find \( \dfrac{dy}{dx}\)
\(12x^2 - 8x + 6\)
\(y = \dfrac{7}{x^{8}} - 3\sqrt[4]{x}\)
Find \( \frac{dy}{dx}\)
\(-\frac{56}{x^{9}} - \frac{3}{4}x^{-\frac{3}{4}}\)
\(y=\sqrt{2x^4-8x}\)
Find \( \dfrac{dy}{dx}\)
\((4x^3-4)(2x^4-8x)^{-\frac{1}{2}}\)
\(y=x \tan x\)
Find \( \dfrac{dy}{dx}\)
\(tanx+\frac{x}{cos^2x}\)
\(y=\frac{x}{\sin x}\)
Find \( \dfrac{dy}{dx}\)
\(\frac{(sinx-xcosx)}{sin^2x}\)
Find the equation of the tangent to the curve:
\(y = -4x^2 + 8x - 5\)
where \(x = -1\)
\(y = 16x - 1\)
Find the equation of the normal to the curve:
\(y = -x^2 + 4x + 2\)
where \(x = 1\)
\(y = 5\frac{1}{2} - \frac{x}{2}\)
\(y =6x^2 - 12x + 4\)
Find \( \int y \quad dx\)
\(2x^3 - 6x^2 + 4x+c\)
A game is played 10 times and the probability of winning is 0.5. Calculate the probability of winning exactly 4 times. 0.205
Make up a maths question using this:
\(^nC_r=\dfrac{n!}{r!(n-r)!}\)
Combinations
(from n choose r)
What letter is this?


Two terms of an arithmetic sequence:
\(u_{7} = 67\)
\(u_{15} = 147\)
Find the sum of the first 39 terms.7683
Find the equations of the asymptotes of:
\(y=\dfrac{2x+5}{2x+3}\)
\(x=-\frac{3}{2},y=1\)
In the triangle ABC,
AB = 9.1cm.
BC = 7.9cm.
CA = 8.9cm.
Find angle CÂB.
52.0°
Evaluate:
$$\sum_{n=4}^{7} 5n-3$$
98
\(f(x)=-7x^2+8x+1\)
What is the value of the discriminant and what does it indicate?
92, Two distinct roots
\(f(x)=x^2+6x-8\)
By completing the square find the coordinates of the vertex.
(-3, -17)
Express \(\log_2(32)\) in terms of a log to base 4.
\( 10\log_4(2) \text{ or } \log_4(1024) \)
Find the integral:
\(\int \dfrac{5x}{x^2-3} \;dx\)
\(\frac{5}{2} \ln(x^2-3)+c\)
Find the equation of the straight line that passes through:
(-2, -4) and (8, 16)
\(y=2x+0\)
Find the inverse of the function \(f\):
\(f(x)=\frac{\sqrt{x-5}}{5}\)
\(25x²+5\)
\(\text{Find }f(x) \text{ if} \\ f(a^3)=2a^6 \\\)
\(f(x)=2x^2\)
Write in standard form:
\(a \times 10^p \times b\times 10^q\)
where \(a \times b \) is a single digit number \((1 \le ab \lt 10)\)
\(ab\times10^{p+q}\)
Draw a rough sketch of
\(y=x^2+7x\)
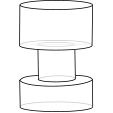
Sketch a height-time graph as this jar is filled.
Without a calculator find the exact value of
$$\cos{\frac{\pi}{4}} + \sin{45°}$$\(\sqrt{2}\)
Without a calculator find the exact value of
$$\tan{\dfrac{19\pi}{3}}$$\(\sqrt{3}\)
Solve:
\( g-7h-7i=-53 \\ 2g-2h+i= 2\\ 5g+3h+i = 31\)
g = 3, h = 4, i = 4
Find the area of a sector with radius 4.5cm and angle \( \frac{\pi}{4}\)
🍕
7.95cm2
In how many ways can 11 different books be arranged on a shelf if 4 of them must be together?
967680
Find the equations of the asymptotes of:
$$y=\dfrac{8x^2-19x-15}{1-2x}$$x=1/2,y=15/2-4x
Evaluate:
$$ \sum_{k=1}^{8} \left( \dfrac12 \right)^{k-3} $$
7.97
Find the first 4 terms in the expansion of:
\((1+2x)^{\frac12}\)
\(1+x-\frac{x^2}{2}+\frac{x^3}{2}\)
Evaluate:
\(\int^{4}_{0} e^x dx\)
\(e^{4}- 1 \approx 53.6\)
Tin A contains 4 red balls and 5 green balls. Tin B contains 8 red balls and 10 green balls. A dice is thrown and if the score is less than 3 a ball is selected from tin A; otherwise a ball is selected from tin B. Given that the ball selected was red, calculate the probability that it came from tin B.
\(\frac{2}{3}\)
Find the point of intersection of \(L_1\) and \(L_2\) if:
\(L_1: \quad \dfrac{x+4}{3} = y-2 = \dfrac{z+1}{2} \)
\(L_2: \quad x = \dfrac{y-5}{2} = \dfrac{-z-1}{2} \)
\( (-1,3,1) \)
Simplify
$$ (3+i)^{-2} $$
\(\frac{2}{25}-\frac{3}{50}i\)
Evaluate:
\(\int x\cos{x}\; dx\)
\(xsinx+cosx+c\)
Simplify:
$$\dfrac{\sin^2{x}-1}{\cos{x}}$$\(-\cos{x}\)
$$ \DeclareMathOperator{cosec}{cosec} $$Find the volume of revolution when \(y=\sqrt{x}\) is rotated about the y-axis for \(1 \le y \le 4\)
\(\frac{1023\pi}{5}\) cubic units
What is the difference between a permutation and a combination?
Permutations consider order; combinations do not.
Show how the first four terms of the Maclaurin series are obtained for
\(f(x) = \sin(x)\)
\(x - \frac{x^3}{6} + \frac{x^5}{120} - \frac{x^7}{5040}\)
Expand and simplify:
$$ (i-\sqrt{3})^5 $$
\(16\sqrt{3} + 16i \\ \text{or } 16(\sqrt{3} + i)\)
5 alphabet blocks A, E, P, R and S are placed at random in a row. What is the likelihood that they spell out either SPEAR or PARSE?
1/60 or 1.67%
Prove by mathematical induction that \( 5^n - 1 \) is divisible by 4 for all natural numbers \( n \)
Show true for n=1, assume true for n=k, prove for n=k+1
Simplify:
$$\sqrt{18}$$
\(3\sqrt{2}\)
Simplify:
$$\dfrac{5}{\sqrt{8}}$$\(\frac{5\sqrt{8}}{8} = \frac{5\sqrt{2}}{4}\)
Simplify
\((3 + 2\sqrt{5})(6 - 3\sqrt{5})\)
\(3\sqrt{5}-12\)
Simplify:
$$\dfrac{8}{3 + \sqrt{6}}$$\(\frac{24 - 8\sqrt{6}}{3}\)
Calculate the standard deviation of the following numbers:
9, 13, 15, 17, 21
4
Write down a summary of your last Maths lesson focussing on what you learnt.
?
Tick (or untick) the boxes above to select the concepts you want to be included in this Starter [untick all]. The display at the top of this page will change instantly to show your choices. You can also drag the panels above so that the questions are ordered to meet your needs.
* Topics shown with an asterix are on the IB Higher Level syllabus but not included in the Standard Level syllabus.
This Starter is called Refreshing Revision because every time you refresh the page you get different revision questions.
Regularly use this Starter to keep that important learning from being forgotten. Here is the web address (URL) for the version of this page with your currently selected concepts:
Copy and paste the URL above into your lesson plan or scheme of work.
For more ideas on revision there are plenty of tips, suggestions and links on the Mathematics Revision page.
Answers appear here for Transum subscribers.
Try this Uniqueness Game with your class.
Transum.org/Maths/Game/Uniqueness/Game.asp?Level=8

Teacher:
Scroll down the
page to see how
this Starter can be customised so that it
is just right for
your class.